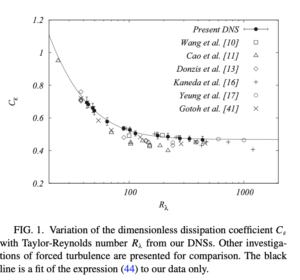
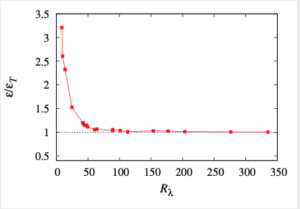
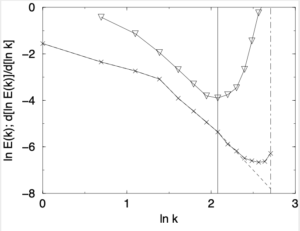
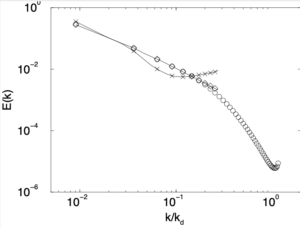
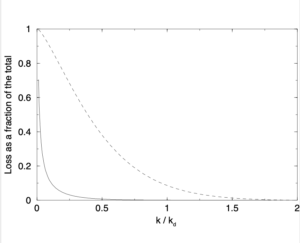
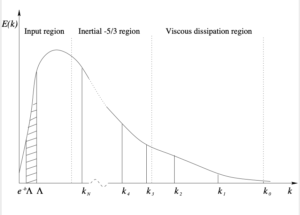
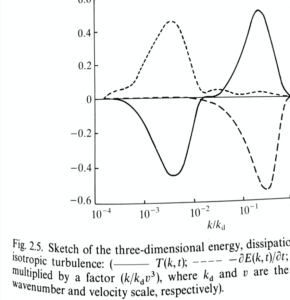
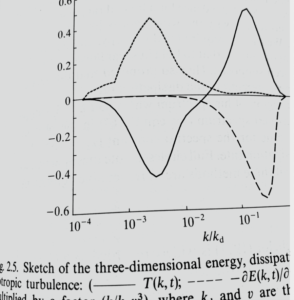
Should theories of turbulence be intelligible to fluid dynamicists?
Should theories of turbulence be intelligible to fluid dynamicists?
One half of the Nobel Prize in physics for 2020 was awarded to Roger Penrose for demonstrating that ‘black hole formation is a robust prediction of the General Theory of Relativity’. While it’s not my field, I do know a little about general relativity; so I had a look at what I could find online. It rapidly became clear to me that in order to understand Penroses’s work in detail, I would have to master a great deal of mathematics – topology in particular – which is unfamiliar to me. This would mean giving up everything else for a substantial period of time and that just wouldn’t make sense. So, despite knowing the basic equations of general relativity (for a simple, yet reasonably complete introduction, see reference [1]), I just have to take the word of other people that it all makes sense.
So what about relativistic quantum field theories, derived from the Navier-Stokes equations? Well, starting with Kraichnan, Wyld and Edwards in the early 1960s and leading up to my own LET theory [2], there exists a moderately successful class of statistical theories of turbulence which are essentially based on quantum field theory. Unfortunately, I would assume that many (most?) fluid dynamicists are as unfamiliar with the background to these as I am with the methods of Penrose in demonstrating that general relativity implies the existence of black holes. Although at least I hope that I belong to the same ‘culture’ as Penrose, in the sense that I appreciate the significance of what he has done and also why he has done it.
The question of how understandable (to turbulence researchers) statistical theories should be, was raised in lecture notes entitled ‘Problems and progress in the theory of turbulence’ [3] by Philip Saffman. In these he wrote down his list of the properties a theory should have. These were generally unexceptionable and really quite obvious. Indeed, one should perhaps bear in mind that a physicist would be very unlikely to write down a similar list, essentially because they would regard it all as being understood. The point that particularly interests me is that the second item in his list, after ‘Clear physical or engineering purpose’ is ‘Intelligibility’. It is worth quoting exactly what he says about this.
‘Intelligibility means that it can be understood, appreciated and applied by a competent scientist without years of study or familiarity with the jargon and techniques of a narrow speciality.’
Obviously, in view of what I wrote at the beginning of this post, I have a certain amount of sympathy with this view. At the same time, I feel that I should challenge it. The final phrase, which I have emphasised, has a faint flavour of the pejorative about it, particularly when taken in conjunction with his other writings. But we are entitled to ask what he means, by a ‘narrow speciality’.
His concern was with those theories of turbulence which are applications of quantum field theory, a subject that made great advances in the 1940s/50s. But quantum field theory was not a ‘narrow speciality’ in the 1970s; and is even less so today. It is a major discipline worldwide and, if we add in statistical field theory in condensed matter physics, then the activity involved would dwarf all turbulence research by orders of magnitude. Moreover, the theory in these areas is closely linked to the experimental work. There is a vast, and growing, body of work in these areas, so this cannot be seen as a narrow or esoteric activity.
Presumably then, he meant simply the applications to turbulence. For Saffman this boiled down to the work of Kraichnan, so he does not give a balanced or scholarly view of this field. Indeed, he does not cite any of the relevant papers by Kraichnan but instead relies on the book by Leslie. It is difficult to see his comments generally as being anything but an expression of frustration that there is an activity going on which he does not understand, combined with a degree of resentment because he felt that his own type of work was somehow being belittled or patronised.
here are other parts of his lecture notes that I value, such as his criticism of Kolmogorov’s 1962 ‘refined theory’; and the general tone of the lectures is undoubtedly stimulating. But although Philip Saffman is no longer here to speak for himself, I still think that his views about fundamental approaches to turbulence should be challenged, if only because similar views seem to be quite widespread today. I am occasionally surprised by how glibly members of the turbulence community are prepared to write off renormalization methods, with phrases such as ‘everyone knows that Kraichnan’s theory is wrong and no one bothers about it anymore’. Well life is so much easier if you pass up on the challenges. But to such people, I would address the question: what have you got to put in its place?
In the mid-1970s, when Saffman was writing, the situation was very different from that today. The basic idea of the LET theory was put forward by me in 1974, incidentally offering a fundamental reason for the failure of the Edwards theory and other cognate theories, including Kraichnan’s. Since then the LET theory has been developed and extensively computed and compared to other theories. I have also published three books, all intended to make such theories more accessible to non-physicists. Two are on turbulence and one on renormalization methods; and their titles can be found in the list of my publications in this blog. So I would like to answer my own question by saying that turbulence theories are intelligible to fluid dynamicists, provided that they are open minded and are prepared to make a bit of an effort. That’s what I would like to say but I have to make one caveat. There are theories, supposedly of turbulence, which are simply a relabelling of text book equations from quantum field theory with variables appropriate to turbulence. Yet such theories do not engage with the existing body of work or explain how they solve problems that others encountered. They used to appear in obscure journals of the old Soviet Union, but now they appear in the learned journals of the west. It appears that the authors do not understand that their work is unsound or perhaps do not care. I intend to write on the subject of Fake Theories (don’t know what put that idea in my head!) but as a topic it presents its difficulties.
Lastly, for completeness, I should mention that there is a class of theories based on the use of Lagrangian coordinates. A recent development in this type of theory also presents a decent and balanced review of other work in the field [4]. I also intend to write about Lagrangian theories in a future post.
[1] W. D. McComb. Dynamics and Relativity. Oxford University Press, 1999.
[2] W. D. McComb and S. R. Yoffe. A formal derivation of the local energy transfer (LET) theory of homogeneous turbulence. J. Phys. A: Math. Theor., 50:375501, 2017.
[3] P. G. Saffman. Problems and progress in the theory of turbulence. In H. Fiedler, editor, Structure and Mechanisms of Turbulence II, volume 76 of Lecture Notes in Physics, pages 273-306. Springer-Verlag, 1977.
[4] Makoto Okamura. Closure model for homogeneous isotropic turbulence in the Lagrangian specification of the flow field. J. Fluid Mech., 841:133, 2018.