Turbulence as a quantum field theory: 1

In the late 1940s, the remarkable success of arbitrary renormalization procedures in quantum electrodynamics in giving an accurate picture of the interaction between matter and the electromagnetic field, led on to the development of quantum field theory. The basis of the method was perturbation theory, which is essentially a way of solving an equation by expanding it around a similar, but soluble, equation and obtaining the coefficients in the expansion iteratively.

As a result of these successes, perturbation theory became part of the education of every physicist. Indeed, it is not too much to say that it is part of our DNA. Yet, a few years ago, when I looked at the website of an applied maths department, they had a lengthy explanation of what perturbation theory was, as they were using it on some problem. One simply couldn’t imagine that, on a physics department website, and it illustrates the cultural voids between different disciplines in the turbulence community. For instance, I used to hear/read comments to the effect that ‘isotropic turbulence had been studied for its potential application to shear flows, but this proved not to be the case and now it was of no further interest.’ From a physicist’s point of view, the reason for studying isotropic turbulence is the same as the motivation for being the first to climb Everest. Because it is there! But, interestingly, the study of isotropic turbulence has increased in recent years, driven by the growth of direct numerical simulation of the equations of motion as a discipline in its own right.

However, back to the sixties. The idea of applying these methods to turbulence caught on, and for a while things seem to have been quite exciting. In particular, there were the pioneering theories of Kraichnan, Edwards and Herring. There was also, the formalism of Wyld, which was the most like quantum field theory. At this point, I know from long and bitter experience that there will be wiseacres muttering ‘Wyld was wrong’. They won’t know what exactly is wrong, but they will be quoting a well-known later formalism by Martin, Siggia and Rose. In fact it has recently been shown that the two formalisms are compatible, once some simple procedural changes have been made to Wyld’s approach [1].

We will return to Wyld in a later post (and also to the distinction between formalisms and theories). Here we want to take a critical look at the underlying physics of applying the methods of quantum field theory to fluid turbulence. It is one thing to apply the iterative-perturbative approach to the Navier-Stokes equations (NSE), and another to justify the application of specific renormalization procedures to a macroscopic phenomenon in classical physics. So, let’s begin by formulating the problem of turbulence for this purpose, in order to see whether the analogy is justified.

We consider a cubical box of side $L$, occupied by a fluid which is stirred by random forces with a multivariate-normal distribution and with instantaneous correlation in time. This condition ensures that any correlations which arise in the velocity field are due to the NSE. It also is known as the white noise condition and allows us to work out the rate at which the forces do work on the fluid in terms of the autocorrelation of the random forces, which is part of the specification of the problem. (Occasionally one sees it stated that the delta-function autocorrelation in time is needed for Galilean invariance. I must say that I would like to see a reasoned justification for that statement.)

By expanding the velocity field (and pressure) in Fourier series, we can study the NSE in wavenumber $k$ space. It is usual nowadays to proceed immediately to the limit $L \rightarrow \infty$ and make use of the Fourier integral representation. It is important to note, that this is a limit. It does not imply that there is a quantity $\epsilon = 1/L = 0$. It does however imply that all our procedures and results must be independent of $L$. Then the problem may be seen as one of strong nonlinear coupling, due to the form of the nonlinear term in wavenumber space.

Strong nonlinear coupling? Well that’s the conventional view and it is certainly not wrong. But let’s not be too glib about this. It is well known, and probably has been since at latest the early part of the last century, that making variables non-dimensionless on specific length- and velocity-scales results in a Reynolds number appearing in front of the nonlinear term as a prefactor. Expressing, this in terms of quantum field theory, the Reynolds number plays the part of the coupling constant. In quantum-electrodynamics, the coupling constant is the fine-structure constant with a value of about $1/137$, and thus provides a small parameter for perturbation expansion. While the resulting series is not strictly convergent, it does give answers of astonishing accuracy. It is equally well known that attempting perturbation theory in fluid dynamics is unwise for anything other than creeping flow, where the Reynolds number is small. So applying perturbation theory to turbulence looks distinctly unpromising.

There is also the basic phenomenology of turbulence which we must take into account. The stirring motion of the forces will produce fluid velocities with normal (or Gaussian) distributions. Then the effect of the nonlinear coupling is to generate modes with larger values of wavenumber than those initially stirred. This is accompanied by the transfer of energy from small wavenumbers to large, and if left to carry on would lead to equipartition for any finite set of modes, albeit with the total energy increasing with time. This assumes the imposition of a cut-off wavenumber, but in practice the action of viscosity is symmetry-breaking, and the kinetic energy of turbulent motion leaves the system as heat. The situation is as shown in the sketch which, despite our restriction to isotropic turbulence in a box, is actually quite illustrative of what goes on in many turbulent flows.

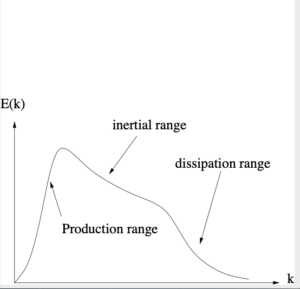
Various characteristic scales can be defined, but the most important is the Kolmogorov dissipation wavenumber, thus: $k_d=(\varepsilon /\nu_0^3)^{1/4}$, which gives the order of magnitude of the wavenumber at which the viscous effects begin to dominate. For the application of renormalized perturbation theory (which we will discuss in a later post), this phenomenology is important for assessment purposes. However, when we look at the later introduction of renormalization group theory, we have to consider this picture in rather more detail. We will do that in the next post.

[1] A. Berera, M. Salewski, and W. D. McComb. Eulerian Field-Theoretic Closure Formalisms for Fluid Turbulence. Phys. Rev. E, 87:013007-1-25, 2013.

Is there an alternative infinite Reynolds number limit?

I first became conscious of the term dissipation anomaly in January 2006, at a summer school, where the lecturer preceding me laid heavy emphasis on the term, drawing an analogy with the concept of anomaly in quantum field theory, as he did so. It seemed that this had become a popular name for the fact that turbulence possesses a finite rate of dissipation in the limit as the viscosity tends to zero. I found the term puzzling, as this behaviour seemed perfectly natural to me. At the time it occurred to me that it probably depended on how you had first met turbulence, whether the use of this term seemed natural or not. In my case, I had met turbulence in the form of shear flows, long before I had been introduced to the study of isotropic turbulence in my PhD project.

Back in the real world, the experiments of Osborne Reynolds were conducted on pipe flow in the late 1890s, and this line of work was continued in the 1930s and 1950s by (for example) Nikuradse and Laufer [1]. This led to a picture where turbulence was seen as possessing its own resistance to flow. The disorderly eddying motions were perceived to have a randomizing effect analogous to, but much stronger than, the effects of the fluid’s molecular viscosity. This in turn led to the useful but limited concept of the eddy viscosity. As the Reynolds number was increased, the eddy viscosity became dominant, typically being two orders of magnitude greater than the fluid viscosity.

In principle, there are three alternative ways of varying the Reynolds number in pipe flow, but in practice it is just a matter of turning up the pump speed. Certainly no one would try to do it by decreasing the viscosity or increasing the pipe diameter. In isotropic turbulence, the situation is not so straightforward, as we use forms of the Reynolds number which depend on internal length and velocity scales. Indeed the only unambiguous characteristic which is known initially is the fluid viscosity.

An ingenious way round this was given by Batchelor (see pp 106 – 107, in [2]), who introduced a Reynolds number for an individual degree for freedom (i.e. wave-number mode) as $R(k) = [E(k)]^{1/2}/\nu k^{1/2}$, in terms of the wavenumber spectrum, the viscosity and the wave-number of that particular degree of freedom. He argued that the effect of decreasing the viscosity would be to increase the dominance of the inertial forces on that particular mode, so that the region of wave-number space which is significantly affected by viscous forces moves out towards $k=\infty$. He concluded: `In the limit of infinite Reynolds number the sink of energy is displaced to infinity and the influence of viscous forces is negligible for wave-numbers of finite magnitude.’ A similar conclusion was reached by Edwards from a consideration of the Kolmogorov dissipation wave-number [1], who showed that the sink of energy at infinity could be represented by a Dirac delta function.

It is perhaps also worth mentioning that the use of this local (in wave-number) Reynolds number provides a strength parameter for the consideration of isotropic turbulence as an analogous quantum field theory [3].
Evidently the conclusion that the infinite Reynolds limit in isotropic turbulence corresponds to a sink of energy at infinity in $k$-space seems to be well justified. Nevertheless, this use of the value infinity in the mathematical sense is only justified in theoretical continuum mechanics. In reality it cannot correspond to zero viscosity. It can be shown quite easily from the phenomenology of the subject that the infinite Reynolds number behaviour of isotropic turbulence can be demonstrated asymptotically to any required accuracy without the need for zero viscosity. We shall return to this in a later post.

1. W. D. McComb. The Physics of Fluid Turbulence. Oxford University Press, 1990.
2 G. K. Batchelor. The theory of homogeneous turbulence. Cambridge University Press, Cambridge, 1st edition, 1953.
3. W. David McComb. Homogeneous, Isotropic Turbulence: Phenomenology, Renormalization and Statistical Closures. Oxford University Press, 2014.

What relevance has theoretical physics to turbulence theory?

The question is of course rhetorical, as I intend to answer it. But I have to pause on the thought that it is also unsatisfactory in some respects. So why ask it then? Well my reply to that is that various turbulence researchers have over the years in effect answered it for me. Their answer would be none at all! In fact, in the case of various anonymous referees, they have often displayed a marked hostility to the idea of theoretical physicists being involved in turbulence research. But the reason why I find it unsatisfactory is that it seems to assume that turbulence theory is not part of theoretical physics, whereas I think it is; or, rather, it should be. So let’s begin by examining that question.

As is well known, the fundamental problem of turbulence is the statistical closure problem that is posed by the hierarchy of moments of the velocity field. Well, molecular physics has the same problem when the molecules interact with each other. This takes the form of the BBGKY hierarchy, although this is expressed in terms of the reduced probability distribution functions. If we consider the simpler problem, where molecules are non-interacting hard spheres, then we have classical statistical physics. In these circumstances we can obtain the energy of the system simply by adding up all the individual energies. The partition function of the system then factorizes, and we can obtain the system free energy quite trivially. However, if the individual molecules are coupled together by an interaction potential, then this factorization is no longer possible as each molecule is coupled to every other molecule in the system. So it is for turbulence, if we work in the Fourier wavenumber representation, the modes of the velocity field are coupled together by the nonlinear term in the velocity field, thus posing an example of what in physics is called the many-body problem.

One could go on with other examples in microscopic physics, for example the theory of magnetism which involves the coupling together of all spins on lattice sites, but it really boils down to the fact that the bedrock problem of theoretical physics is that of strong-coupling. And turbulence formulated in $k$-space comes into that category. The only difference is, that turbulence is mainly studied by engineers and applied scientists, while theorists mostly prefer to study what they see as more fundamental problems, even if these studies become ever more arid for lack of genuine inspiration or creativity. But as a matter of taxonomy, not opinion, turbulence should belong to physics as an example of the many-body problem.

Now let’s turn to our actual question. We can begin by noting that we are talking about insoluble problems. That is, there is no general method of obtaining an exact solution. We have to consider approximate methods. First, there is perturbation theory, which relies on (and is limited by) the ability to perform Gaussian functional integrals. Secondly, there is self-consistent field theory. Both of these rely, either directly or indirectly, on the concept of renormalization. In molecular physics, this involves adding some of the interaction energy to the bare particle, in order to create a dressed particle, also known as a quasi-particle. Such quasi-particles do not interact with each other and so the partition function can be evaluated by factorization, just as in the ideal-gas case. In the case of turbulence, it is probably quite widely recognized nowadays that an effective viscosity may be interpreted as a renormalization of the fluid kinematic viscosity. However, it should be borne in mind that the stirring forces and the interaction strength may also require renormalization.

There is no inherent reason why the subject of statistical turbulence theory should be mysterious and I intend to post short discussions of various aspects. Not so much maths, as `good versus bad’ or `justified versus unjustified’; plus tips on how to use some common sense reasoning to cut through the intimidatingly complicated mathematics and (in some cases self-important pomposity) of some theories which are not really new turbulence theories but merely text-book material from quantum field theory in which variables have been relabelled, but the essential difficulties of extending to turbulence have not been tackled.

The Kolmogorov `5/3’ spectrum and why it is important

An intriguing aspect of the Kolmogorov inertial range spectrum is that it was not actually derived by Kolmogorov. This fact was unknown to me when, as a new postgraduate student, I first encountered the `5/3’ spectrum in 1966. At that time, all work on the statistical theory of turbulence was in spectral or wavenumber ($k$) space , and the Kolmogorov form was seen as playing an important part in deciding between alternative theoretical approaches.

As is well known nowadays, in 1941 Kolmogorov derived power-law forms for the second- and third-order structure functions in $r$ space. In the same year, it was Obukhov [1] who worked in $k$ space, introducing the energy flux through wavenumber as the spectral realization of the Richardson-Kolmogorov cascade, and making the all-important identification of the scale-invariance of the energy flux as corresponding to the Kolmogorov picture for real space. It is usual nowadays to denote this quantity by $\Pi(k)$, and in this context scale-invariance means that it becomes a constant, independent of $k$. For stationary turbulence that constant is the dissipation rate. Obukhov did actually produce the `5/3’ law, but this involved additional hypotheses about the form of an effective viscosity, so it was left to Onsager in 1945 [2] to combine simple dimensional analysis with the assumption of scale-invariance of the flux to produce a spectral form on equal terms with Kolmogorov’s `2/3’ law for $S_2(r)$. This work was discussed (and in effect) disseminated by Batchelor in 1947 [3], and later in his well-known monograph. Curiously enough, in his book, Batchelor only discussed the spectral picture, having discussed only the real-space picture in [3]. This is something that we shall return to in later posts. But it seems that the effect was to establish the dominance of the spectral picture for many years.

In the early sixties, there was considerable excitement about the new statistical theories of turbulence, but when Grant, Stewart and Moilliet published their experimental results for spectra, which extended over many decades of wavenumber, it became clear beyond doubt that the Kolmogorov inertial-range form was valid and that the theories of Kraichnan and Edwards were not quite correct. We will write about this separately in other posts, but for me in 1966 the challenge was to produce an amended form of the Edwards theory which would be compatible with the `5/3’ spectrum. This, in other words, was a restatement of the turbulence closure problem. It is one that I have worked on ever since.

This is not an easy problem and progress has been slow. But there has been progress, culminating in McComb & Yoffe (2015): see #3 of my recent publications. However, over the years, beginning in the late 1970s, this work has increasingly received referee reports which are hostile to the very activity and which assert that the basic problem for closures is not to obtain $k^{-5/3}$ but rather to obtain a value for $\mu$, where the exponent should be $-5/3 + \mu$, due to intermittency corrections. Unfortunately for this point of view, the so-called intermittency correction $\mu$ comes attached to a factor $L$, representing the physical size of the system. This means that the limit $L \rightarrow \infty$ does not exist, which is something of a snag for the modified Kolmogorov theory.

We shall enlarge on this elsewhere. For the moment it is interesting to note that the enthusiasm for intermittency corrections arose from the study of structure functions and in particular their behaviour with increasing order. This became a very popular field of research throughout the 1980s/90s and threatened to establish a sort of standard model, from which no one was permitted to dissent. Fortunately, there has been a fight back over the last decade or two, and the importance of finite Reynolds number effects (or FRN) is becoming established. In particular, the group consisting of Antonia and co-workers has emphasised consistently (and in my view correctly) that the Kolmogorov result $S_3 \sim (4/5)r$ (which the Intermittentists regard as exact) is only correct in the limit of infinite Reynolds numbers. At finite viscosities there must be a correction, however small. A similar conclusion has been reached for the second-order structure function by McComb et al (2014), who used a method for reducing systematic errors to show that this exponent too tended to the canonical value in the limit of infinite Reynolds numbers. These facts have severe consequences for the way in which the Intermittentists analyse their data and draw their conclusions.

This leaves us with an interesting point about the difference between real space and wavenumber space. The above comments are true for structure functions, because in $r$-space everything is local. In contrast, the nonlinear energy transfers in $k$-space are highly nonlocal. The dominant feature in wavenumber space is the flux of energy through the modes, from low wavenumbers to high. The Kolmogorov picture involves the onset of scale invariance at a critical Reynolds number, and the increasing extent of the associated inertial range of wavenumbers as the Reynolds number increases. The infinite Reynolds number limit in $k$-space then corresponds to the inertial range being of infinite extent. At finite Reynolds numbers, it will be of merely finite extent, but there is no reason to believe that there is any other finite Reynolds number correction. I believe that this is more than just a conjecture.

[1]A. M. Obukhov. On the distribution of energy in the spectrum of turbulent flow. C.R. Acad. Sci. U.R.S.S, 32:19, 1941.

[2] L. Onsager. The Distribution of Energy in Turbulence. Phys. Rev., 68:281, 1945.

[3] G. K. Batchelor. Kolmogorov’s theory of locally isotropic turbulence. Proc. Camb. Philos. Soc., 43:533, 1947.

Scientific discussion in the turbulence community.

Shortly after I retired, I began a two-year travel fellowship, with the hope of having interesting discussions on various aspects of turbulence. I’m sure that I had many interesting discussions, particularly in trying out some new and half-baked ideas that I had about that time, but what really sticks in my mind are certain unsatisfactory discussions.

To set the scene, I had recently become aware of Lundgren’s (2002) paper [1] and, having worked through it in detail, I was convinced that it offered a proof that the second-order structure function took the Kolmogorov `2/3’ form asymptotically in the limit of infinite Reynolds numbers. There is of course little or no disagreement about Kolmogorov’s derivation of the `4/5’ law for the third-order structure function. For stationary turbulence, it is undoubtedly asymptotically correct in the infinite Reynolds number limit. But in order to find the second-order form, Kolmogorov had to make the additional assumption that the skewness of the longitudinal derivative became constant in the infinite Reynolds number limit. Introducing the skewness $S$ as $S=S_3(r)/S_2(r)^{3/2}$, and substituting the `4/5’ law for $S_3$, results in the well-known form $S_2(r)=(-4/5S)^{2/3}\varepsilon^{2/3}r^{2/3}\equiv C_2\varepsilon^{2/3}r^{2/3}$. Numerical results do indeed suggest that the skewness becomes independent of the Reynolds number as the latter increases, but it remains a weakness of the theory that this assumption is needed.

Lundgren [1] started, like Kolmogorov, from the Karman-Howarth equation (KHE), and did the following. He put the KHE in dimensionless form by a generic change of variables based on time-dependent length and velocity scales, $l$ and $u$. He then chose to examine: first, Von Karman scaling; and secondly, Kolmogorov scaling, with appropriate choices for $l$ and $u$. In both cases, he solved for the scaled second-order structure function by a perturbation expansion in inverse powers of the Reynolds number. He then employed the method of matched asymptotic expansions which recovered the Kolmogorov form for $S_2$. The `4/5’ law was also recovered for $S_3$, both results naturally following in the large Reynolds number limit. A more extensive account of this work can be found in Section 6.4.6 of my 2014 book.

Before setting off on my travels, I consulted a colleague who, although specializing in soft matter, had some familiarity with turbulence. To my surprise he seemed quite unenthusiastic about this work. He said something to the effect that it was a pity that Lundgren had to assume the same scaled form for both the second-order and the third-order structure functions. Now, on reflection I saw that this was nonsense. All Lundgren did was introduce a change of variables: this is not an assumption; it merely restates the problem, as it were. Secondly, the basic Kolmogorov theory deals with the probability distribution functional, and this means that all the moments (and hence structure functions) will be affected in the same way by any operation on it [2].

On the first of my visits, I began to discuss this with Professor X, who seemed very sceptical at first, then his comments seemed increasingly irrelevant, then he realised that he was thinking of an entirely later piece of work by Lundgren. At that point the discussion fizzled out.

On a later visit to a different university, at an early stage in the discussion with Professor Y, I commented that the method relied on the fact that the Karman-Howarth equation was local in the variable $r$. To which he swiftly replied: `Yes Tom does have to assume that.’ That effectively brought things to a close, because once again we are faced with nonsense. In fact this particular individual seems to believe that the existence of an energy cascade implies that the KHE is nonlocal! But of course the nonlocalness is confined to the Lin equation in wavenumber space.

On a later occasion, I tried to bring the subject up again, but no luck. He said: `Tom just makes the same assumptions as Kolmogorov did. So there is nothing new.’ At this point I finally gave up. However, as we have just seen, Kolmogorov has to assume that the skewness $S$ becomes constant as the Reynolds number increases. In contrast, the Lundgren analysis actually shows that this is so. In addition, it also provides a way of assessing systematic corrections to the `4/5’ law at large but finite Reynolds numbers.

The basic theoretical problems in turbulence are very hard and perhaps even impossible to solve, in a strict sense. However, the fact that lesser problems of phenomenology are plagued by controversy, with issues remaining unresolved for decades, seems to me to be a matter of attitude (and culture) that leads to a basic lack of scholarship. I think we need to trade in the old turbulence community and get a new one.

[1]Thomas S. Lundgren. Kolmogorov two-thirds law by matched asymptotic expansion. Phys. Fluids, 14:638, 2002.

[2] I have to own up to an error here. For years I argued that only the second- and third-order structure functions were involved in Kolmogorov and hence conclusions based on higher-order moments were irrelevant. Then (quite recently!) I noticed in a paper by Batchelor the comment that as the hypotheses were for the pdf, they automatically applied to moments of all orders.